问题及解答

证明:

(1) 当 $x > 1$ 时, 

\[
\arctan x-\arctan\frac{1+x}{1-x}=\frac{3\pi}{4},
\]

(2) 当 $x < 1$ 时, 

\[
\arctan\frac{1+x}{1-x}-\arctan x=\frac{\pi}{4}.
\]

这里还给出了关于(2)的两个几何证明.

令 $\alpha=\arctan\frac{1+x}{1-x}$, $\beta=\arctan x$. 则

\[
\begin{split}
\tan(\alpha-\beta)&=\frac{\tan\alpha-\tan\beta}{1+\tan\alpha\cdot\tan\beta}\\
&=\frac{\frac{1+x}{1-x}-x}{1+\frac{1+x}{1-x}\cdot x}\\
&=\frac{1+x-x(1-x)}{1-x+x(1+x)}\\
&=\frac{1+x^2}{1+x^2}\\
&=1.
\end{split}
\]

故 $\alpha-\beta=\frac{\pi}{4}+k\pi$, $k$ 是某个整数.

注意到

\[
\frac{1+x}{1-x}-x < 0 \Leftrightarrow \frac{1+x-x(1-x)}{1-x} <0 \Leftrightarrow \frac{1+x^2}{1-x} < 0 \Leftrightarrow 1-x <0
\]

分情况讨论:

(1) $\frac{1+x}{1-x}-x < 0$, 即 $x > 1$ 时.

此时

\[
\frac{1+x}{1-x} < -1
\]

从而

\[
\pi=\frac{\pi}{2}-(-\frac{\pi}{2}) > \arctan x-\arctan\frac{1+x}{1-x} > \frac{\pi}{4}-(-\frac{\pi}{4})=\frac{\pi}{2}
\]

由于 $\beta-\alpha=k\pi-\frac{\pi}{4}$, 故

\[
\frac{\pi}{2} < k\pi-\frac{\pi}{4} < \pi
\] 

因此, $k=1$. 即有

\[
\arctan x-\arctan\frac{1+x}{1-x}=\frac{3}{4}\pi
\]

(2) $\frac{1+x}{1-x}-x > 0$, 即 $x < 1$ 时,

此时 $\frac{1+x}{1-x} > 1$. 于是

\[
0 < \arctan\frac{1+x}{1-x}-\arctan x <\frac{\pi}{2}-0=\frac{\pi}{2}
\]

由于 $\alpha-\beta=k\pi+\frac{\pi}{4}$, 故 $k=0$. 即有

\[
\arctan\frac{1+x}{1-x}-\arctan x=\frac{\pi}{4}.
\]

几何证明1

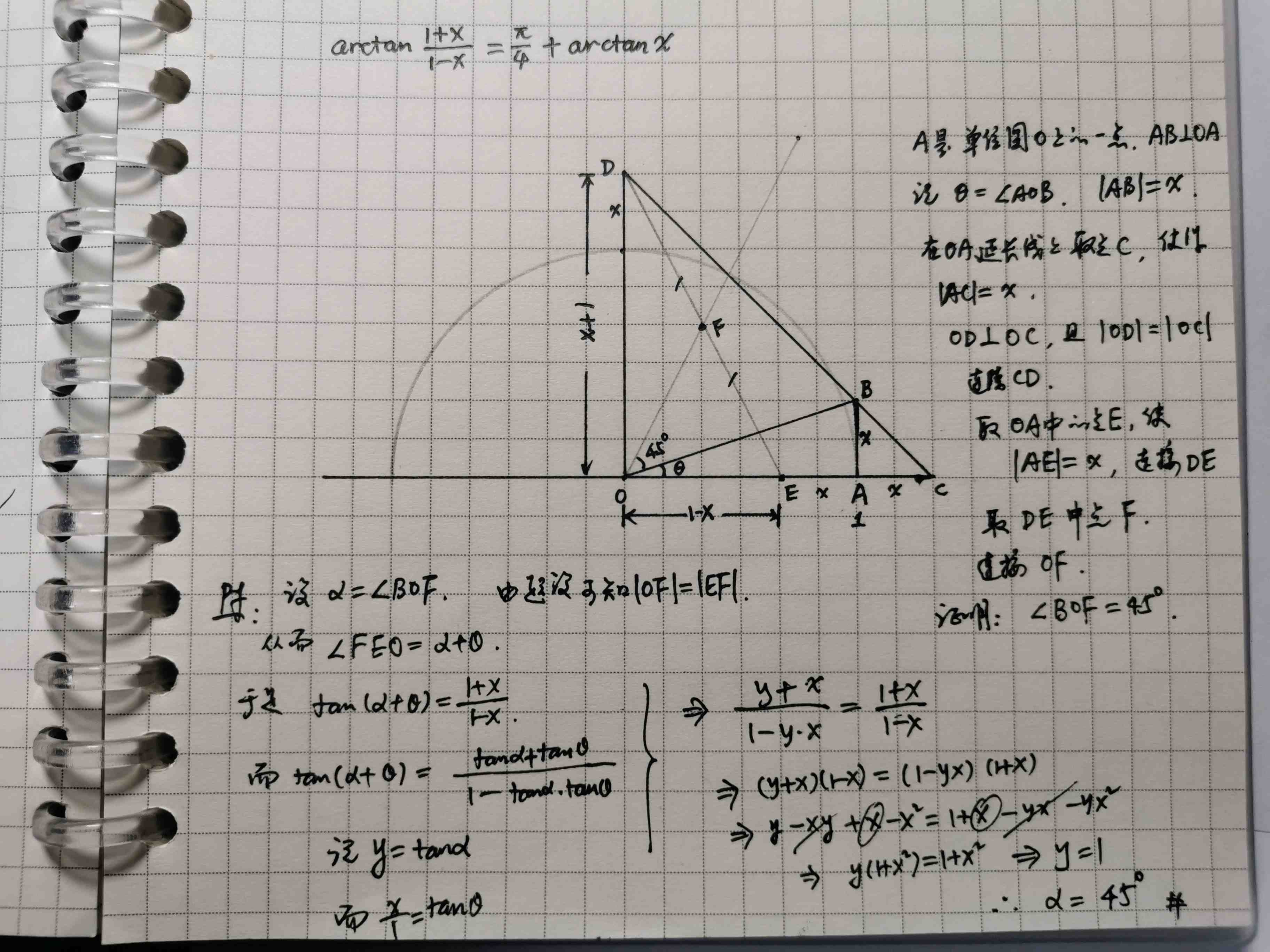
连接 $BF$, 可证明 $BF\perp OF$.

Date:  April 26, 2022.

几何证明 2.  这个很妙的证明是由张影给出的.

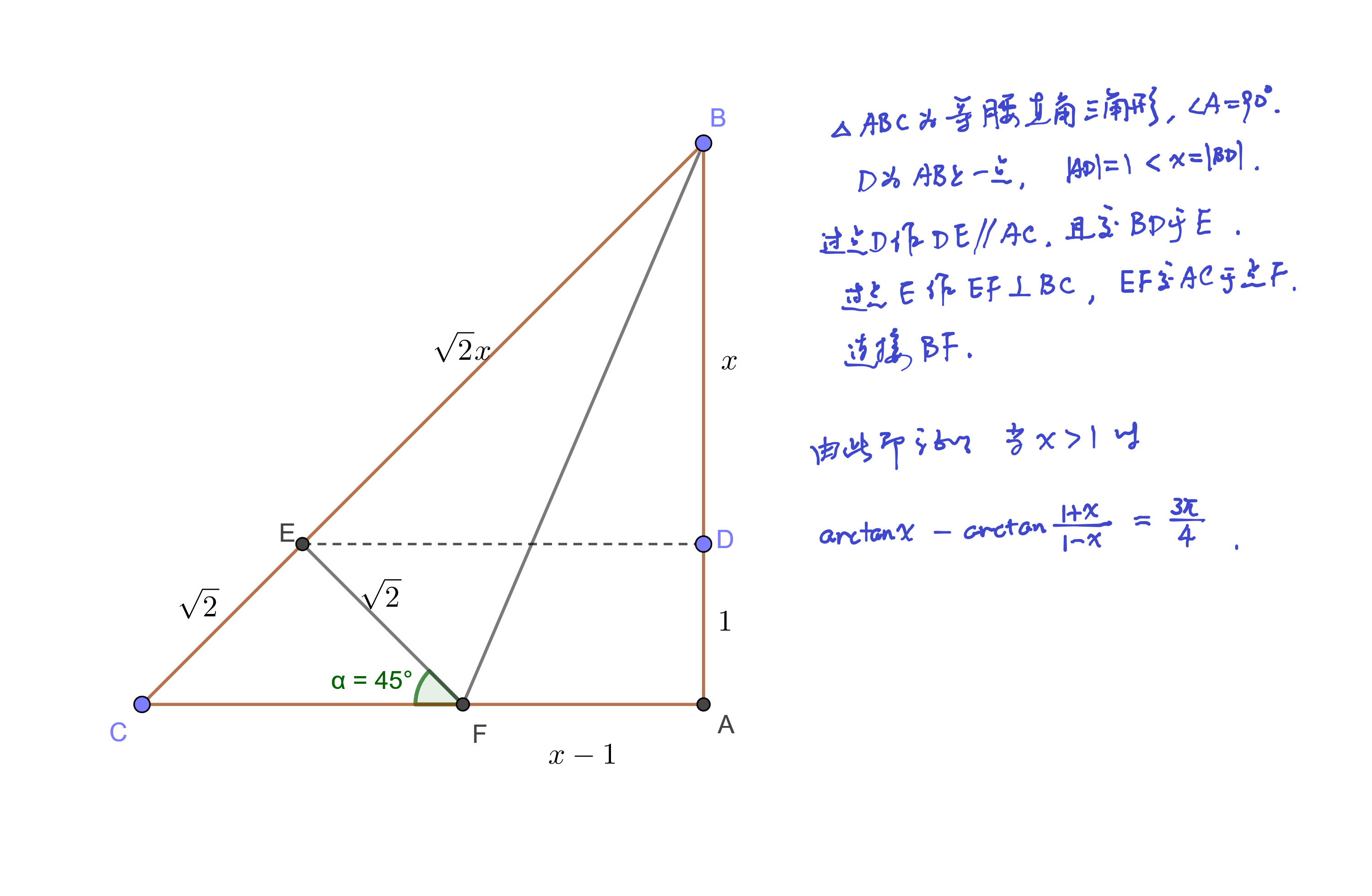
根据张影老师的启发, 对于 $x > 1$ 的情形, 可以用下面的几何方法证明.